Utopi
a

Fundamental diagram and stability of mixed traffic flow considering platoon size and intensity of connected automated vehicles

from Physica A
Zhihong Yao a,b,c, Qiufan Gu a, Yangsheng Jiang a,b,c,∗, Bin Ran d

Abstract

对异质交通流的数学建模，贡献

(1) a greater platoon size leads to the increase of traffic capacity while it is harmful to the maintenance of traffic flow stability;

(2) the platoon size is recommended to be set at 4 to 6 to balance the relationship between traffic capacity and stability;

(3) a more significant platoon intensity can help improve the traffic capacity and stability;

(4) the penetration rate of CAVs has a positive effect on the traffic flow stability until it increases to a certain degree.

Intro

However, CVs strongly depend on a high penetration rate, and AVs cannot predict the driving behavior of multiple vehicles ahead [6,7].

Mixed traffic flow model

T=[tAAtAHtHAtHH]T=\left[\begin{array}{cc} t_{A A} & t_{A H} \\ t_{H A} & t_{H H} \end{array}\right]
tAH(PA,PI)=Pr⁡(An+1=HDV⁡∣An=CAV)={PH(1−PI),PI≥0PH+PI(PH−min⁡{1,PHPA}),PI<0,tAA(PA,PI)=Pr⁡(An+1=CAV∣An=CAV)=1−tAH(PA,PI),\begin{aligned} & t_{A H}\left(P_{A}, P I\right)=\operatorname{Pr}\left(A_{n+1}=\operatorname{HDV} \mid A_{n}=\mathrm{CAV}\right)= \begin{cases}P_{H}(1-P I), & P I \geq 0 \\ P_{H}+P I\left(P_{H}-\min \left\{1, \frac{P_{H}}{P_{A}}\right\}\right), & P I<0,\end{cases} \\ & t_{A A}\left(P_{A}, P I\right)=\operatorname{Pr}\left(A_{n+1}=\mathrm{CAV} \mid A_{n}=\mathrm{CAV}\right)=1-t_{A H}\left(P_{A}, P I\right), \end{aligned}
tHA(PA,PI)=Pr⁡(An+1=CAV⁡∣An=HDV)={PA(1−PI),PI≥0PA+PI(PA−min⁡{1,PAPH}),PI<0,tHH(PA,PI)=Pr⁡(An+1=HDV∣An=HDV)=1−tHA(PA,PI).\begin{aligned} t_{H A}\left(P_{A}, P I\right) & =\operatorname{Pr}\left(A_{n+1}=\operatorname{CAV} \mid A_{n}=\mathrm{HDV}\right)= \begin{cases}P_{A}(1-P I), & P I \geq 0 \\ P_{A}+P I\left(P_{A}-\min \left\{1, \frac{P_{A}}{P_{H}}\right\}\right), & P I<0,\end{cases} \\ t_{H H}\left(P_{A}, P I\right) & =\operatorname{Pr}\left(A_{n+1}=\mathrm{HDV} \mid A_{n}=\mathrm{HDV}\right)=1-t_{H A}\left(P_{A}, P I\right) . \end{aligned}

Cooperative adaptive cruise control mode

{vn(t+Δt)=vn(t)+kpen(t+Δt)+kde˙n(t+Δt)en(t+Δt)=xn−1(t)−xn(t)−l−S0−TAA−intra vn(t).\left\{\begin{array}{l} v_{n}(t+\Delta t)=v_{n}(t)+k_{p} e_{n}(t+\Delta t)+k_{d} \dot{e}_{n}(t+\Delta t) \\ e_{n}(t+\Delta t)=x_{n-1}(t)-x_{n}(t)-l-S_{0}-T_{A A_{-} \text {intra }} v_{n}(t) . \end{array}\right.
an(t)=kp(Δxn(t)−l−S0−TAA_intra vn(t))+kdΔvn(t)Δt+kdTAA_intraa_{n}(t)=\frac{k_{p}\left(\Delta x_{n}(t)-l-S_{0}-T_{A A \_ \text {intra }} v_{n}(t)\right)+k_{d} \Delta v_{n}(t)}{\Delta t+k_{d} T_{A A \_i n t r a}}

Screen Shot 2023-03-06 at 19.28.14

Fundamental diagram

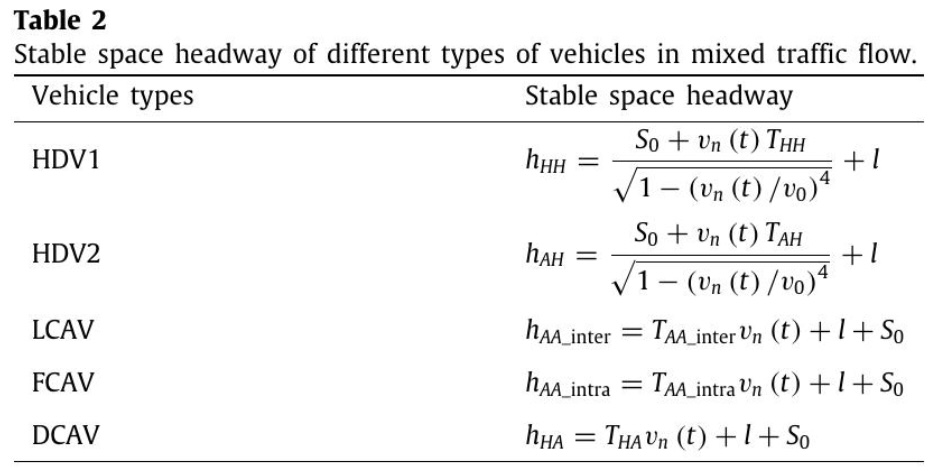
In a word, the space headway in the mixed traffic flow steady state depends on the vehicle types.